The temperature rise of an air-cooled transformer is not only related to the sum of the core loss and the winding copper loss, but also to the area of ​​the radiating surface. As the air flows through the transformer, the temperature of the transformer will decrease, and the degree of decrease is related to the air velocity (in(3)/min).
It is not easy to accurately and systematically calculate the temperature rise of the transformer, but the temperature rise value can be obtained through some empirical curves, and the error is only within 10°C. These curves are based on the concept of thermal impedance of the radiating surface area. The thermal resistance Rt of the heat sink is defined as the temperature rise (usually in °C) brought about by the heat sink per 1W of power dissipated. The relationship between the increase in temperature rise dT and the dissipated power P is: dT=PRt.
Some manufacturers also give the R value of different products, which indirectly indicates that the temperature rise of the outer surface of the magnetic core is the product of Rt and the sum of the core loss and copper loss. Experienced users usually assume that the inner surface is the hottest spot (generally The temperature rise of the central column of the magnetic core is 10-15 °C higher than that of the outer surface of the magnetic core.
The temperature rise is not only related to the area of ​​the radiating surface, but also to the total dissipated power of the magnetic core. The greater the dissipated power of the radiating surface, the greater the temperature difference between the radiating surface and the surrounding air, and the easier the surface is to cool, which means the lower the thermal resistance of the surface.
Therefore, when estimating the temperature rise of the transformer, the total external surface area of ​​the transformer is often regarded as the radiation surface area of ​​an equivalent heat sink. The total external surface area is
(2×width×height＋2×width×thickness＋2×height×thickness)
The thermal impedance of the equivalent heat sink can be corrected according to the total dissipated power (the sum of core loss and copper loss).
The relationship between the thermal impedance of the heat sink and the surface area is shown in Figure (a). This is an empirical curve based on the average value of a large number of different manufacturers, different sizes and different shapes of heat sinks. The thermal impedance values ​​of the 1 W power stage are marked in the figure, which are located on the straight line in the logarithmic coordinate.
Although the thermal impedance of a finned heat sink is somewhat related to the shape of the blades, the gap between the blades, and whether the blades are blackened or aluminized, these are secondary factors. To a certain extent, it can be said that the thermal impedance is completely determined by the area of ​​the radiating surface of the heat sink.
The relationship between thermal impedance and dissipated power as shown in Figure (b) is also given in the product goals of different heat sink manufacturers.
Combining Figures (a) and (b), the relationship between the temperature rise and power loss of different heat sink areas shown in Figure (c) can be obtained. This curve is more direct and convenient to use. It provides the temperature rise values ​​of heat sinks for different heat dissipation areas (diagonals) and dissipating power. The temperature rise on the outer surface of the transformer can therefore be read directly from the graph, since both the total losses and the total radiating surface area are given.
(a) The relationship between the thermal impedance of the heat sink and the surface area
(b) Relationship between thermal impedance and dissipated power
(c) The relationship between the temperature rise and power loss of different heat sink areas

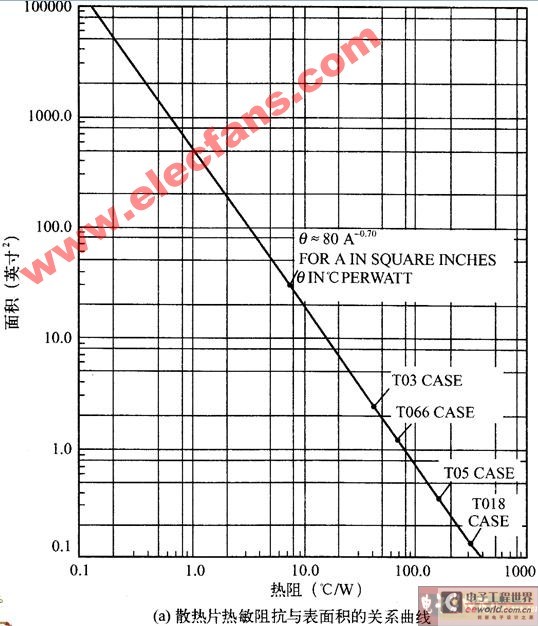
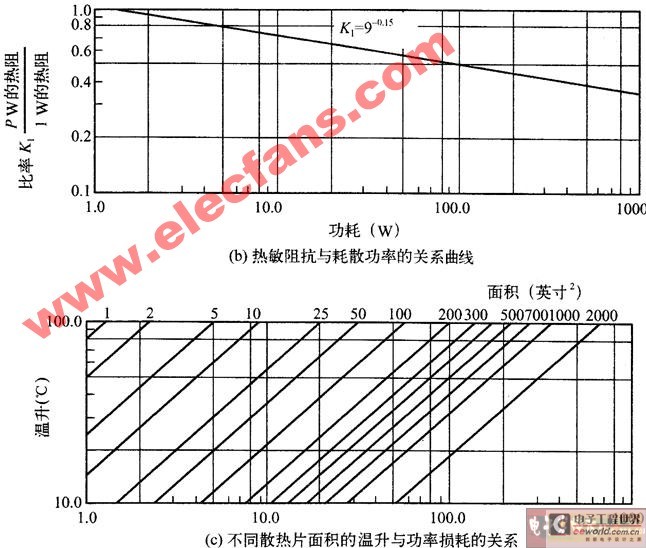
The relationship between temperature rise and power loss for different heat sink areas. Calculate the temperature rise based on the equivalent area of the transformer heat sink.
(This article is transferred from Electronic Engineering World: http://www.eeworld.com.cn/mndz/2011/0810/article_11221.html)